In almost all lamp measurement articles one can find the measured value for Power Factor. In this article I explain more about it.

Definition power factor
The power factor (PF) equals the division between the net consumed power P in Watts by the apparent power S in VoltAmpere:
The PF indicates how efficient the electrical power is consumed by a load (a load can be a computer, a microwave oven, a lamp etc etc). In an ideal case the PF equals 1. And everything lower than 1 indicates that the transport of the needed power is not as efficient.
net consumed power P [W] the net consumed power P is the power consumed by any of the loads and that is where we have to pay the utility company for. An example: an incandescent lamp of 100 W consumes a power P = 100 W. If you leave this lamp on for 10 hours, it has consumed 10 hours x 100 W = 1000 Wh = 1 kWh. In The Netherlands one pays about 18 ct/kWh (price level 2016).
apparent power S [VA] the apparent power S is the sum of net power P AND the power needed to transport the net power P from the grid to the load. So for an incandescent lamp, the load is such that S = P and so to transport the net power P there is nothing more needed and the transport of P is very efficient. This is because an incandescent lamp is purely a resistive load. However when one connects a computer with its power adapter to the grid, of say P = 50 W, then when measuring correctly one will see that there is more power transport needed to get this 50 W across. And as a consequence S is bigger than P. The bigger S, the more energy needs to flow between grid and load in order to transport the power P, and this transport of energy over and back is a waist. This waist is called blind power.
So P is really what is consumed by the load and makes the load work (like a TV that works, a bread toaster, microwave oven, computer etc) and S is the total of P and the blind power together, where blind power indicates energy flowing between load and grid that seems to be needed to transport P.
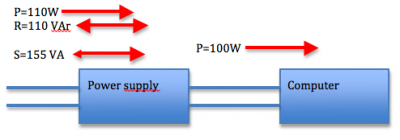
An example: one uses a computer power supply (adapter) to feed a computer with 100 W power. So the transport of new power is 100 W. The power supply itself also needs some enery to do its work, so say that one consumes 10 W. In total 110 W pure power. However the power supply needs some blind power to work well, and one (electrically inclined person) could imagine some energy to magnetize and demagnetize coils, charge and discharge capacitors. This latter blind power is power that flows between the load and the grid, back and forth. It does not add to the pure consumed power since the energy is not consumed but used in order to make the load work as intended, the customer does not have to pay for it, but it has some other disadvantages which will be explained later.
When measuring the current through the device, one would measure more than what one would expect to transport just the net power P. So the bigger the need for blind power, the bigger the current will be through the device. If for this example the blind power would be just as big as the net power, say 110 in value, then the current through the load is the current needed to transport both the net consumed power AND the current for the blind power. The total current would then be 1.4 times bigger which is the square root of 2.
Now in this example the consumed power P is 110 W. The blind power is indicated by R and equals 110 VAr. The arrows indicate that for R this is blind power that flows back and forth between grid and load.
S is the vector sum of both the P and R, hence its value is computed as:
If one would measure the current, then they would find I = S / U = 155 VA / 230 V = 0.67 A. This value of 0.67 A is more than one would expect only from the net consumed power: P / U = 110 W / 230 V = 0.48 A. So the current that flows and can be measured, is in this example 0.67 A and the current that would be needed for only transporting the power P would be 0.48 A. The user pays for the net power, and not the apparent power!
The PF in this example is
This value is less than 1 hence it indicates the current is more than strictly needed.
Impact of the PF
So the PF can be maximally 1, and in that case S = P and hence there is no blind power (R = 0).A private consumer (not a company) does not pay for any blind power, so why would we care? Well, anyway companies that require high amounts of power do pay for blind power, and they do care for that matter.
But there are some negative consequences:
A PF less than 1 means a current higher than needed only for transporting the power P. A higher current however means that there are more losses over the lines and wires in the grid.
Low PF means higher currents means higher losses in the grid and wires
Higher currents also reuslt in higher voltage drop across the wires, resulting in a lower voltage that arrives at the end-user. This has an effect on hair dryers, incandescent lamps etc that work less powerful.
Low PF means higher voltage drop across the wires and lines and hence a lower voltage at the end user
The higher currents due to PF < 1, need still to be delivered by the utility company. They need bigger transformers, generators and wires to generate, deal with and carry safely these higher currents.
Low PF means bigger generators, transformers, and wires to safely carry these higher than strictly needed currents
When an end user has a fuse of 1 x 35 A and has loads that on average have a PF of 0,5, then that means that one can draw only half the net power from its 35 A fused. Since S is 2 x P, and P is the power one needs. So if this end user corrects its PF from 0.5 to 1.0, then this user can draw twice as much. Or the end user pays for a fixed connection with a higher fuse.
Low PF means that the end user can draw less net power from the (fused) grid connection
The losses in the lines in the area of an end user (in the house or small office) are not so high, as the lines are relatively short. But the higher currents due to a low PF result in more heating of the components through which this current flows. It can be the power cords that warm up.
Low PF means higher currents that heat up the power lines and power cords.
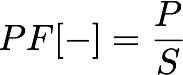
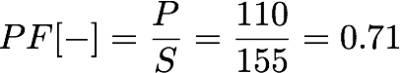





1 reply on “Explaining the power factor”
It would be better if you defined Ar and the actual equations were shown in equation form — i.e. with the equal sign between two mathematical expressions with the definition of each parameter just below the equation. Verbal descriptions of equations are not as effective — especially if the terms are also defined with sentences.